This is my blog on rocket science, astronomy, and spaceflight! I will post interesting space news for your entertainment.
The name of my blog, Delta-V, comes from the basic piece of math from which most rocket science is based; The Tsiolkovsky rocket equation. Which is, quite simply, a way of finding out how far a rocket could get, in a perfect universe. It looks like this:
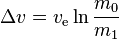
It's named after Konstantin Tsiolkovsky, just so you know.
Don't worry, it's not as bad as it looks.
Let's unpack it. First, moving from the left to the right, we have this symbol: Δv It is pronounced delta-vee. That is the mathematical way of describing change in velocity. If a spacecraft has a Delta-V of 1,200m/s, then, if it was in a gravity free vacuum, it would be traveling at 1,200m/s after it had burned all of it's fuel.
Now, we have the rest of the equation, starting with
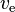, which is the effective exhaust velocity. In other words, how fast the exhaust from the rocket comes out. The faster it comes out, the more efficient the rocket is. Then there's
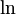, which is the natural logarithm, and that accounts for the fact that the rocket accelerates faster as it uses up fuel.Finally, to factor in the mass fraction, which is how much fuel mass to ship mass there is in the ship. If it's higher, there is more "stuff" to be used for propellant.
If you add it all together, you get a formula which can tell you how far your rocket can go.
And here are some people who explain it better than I:
Wikipedia
Randall Munroe
William Greene
Don Pettit
